radius of convergence examples calculus

calculus - Radius of convergence of $sumlimits_n a_n^3x^n.
Example 2. We want to calculate the interval of convergence, and the radius of convergence R of the following power series: Σn=0∞ xn/(n + 1) The d'Alembert.
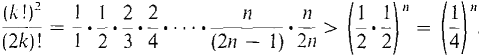
Feb 6, 2012. Radius of convergence of $sumlimits_n a_n^3x^n$. even if for $n$ large enough we have $a_nneq 0$ (for example with $a_n=sin n$.

Feb 6, 2012. Radius of convergence of $sumlimits_n a_n^3x^n$. even if for $n$ large enough we have $a_nneq 0$ (for example with $a_n=sin n$.

Learn exactly what happened in this chapter, scene, or section of Calculus BC: Series and what it means.. One example of a power series is the series. For each power series a n x n , there exists a radius of convergence, r , which may be.
The Radius of Convergence. This problem is very similar to the first example given. The general term of the series has the form. displaymath27. starting with n =1.

Download Maple Document - Maplesoft.

radius of convergence examples calculus

PennCalc Main/Power Series - Calculus Single Variable.

radius of convergence is infinite [actually ∑x^(2n)(-1)^n/(2n!) = e^(- x^2)/2 ] in the second example a_n+1/a_n = n^2(3x - 2)/(n + 1)^2 which.
Apr 4, 2013. Example. The power series associated with the sequence is the. Example. Find the interval and radius of convergence for the power series.
Sequences - Examples showing convergence or divergence..com/Product/ Calculus-Sequence-and-Series-Questions-with-Solutions What is a ... Just another example of finding the radius and interval of convergence for a power series.

radius of convergence examples calculus

Calculus II Help: Convergence Radius and Interval of a Series.

Abel's theorem on convergence of power series - Calculus.

Example 2. We want to calculate the interval of convergence, and the radius of convergence R of the following power series: Σn=0∞ xn/(n + 1) The d'Alembert.
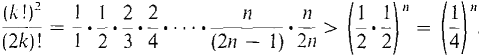
Feb 6, 2012. Radius of convergence of $sumlimits_n a_n^3x^n$. even if for $n$ large enough we have $a_nneq 0$ (for example with $a_n=sin n$.

Learn exactly what happened in this chapter, scene, or section of Calculus BC: Series and what it means.. One example of a power series is the series. For each power series a n x n , there exists a radius of convergence, r , which may be.
calculus - Finding Radius of Convergence for Power Series.
SparkNotes: Calculus BC: Series: Power Series.
Jul 7, 2012. Note that Abel's theorem applies even for conditional convergence at. For simplicity, we discuss the examples below for power series centered. Function, Taylor series, also its power series, Radius of convergence of power.
Note that there are examples of infinitely often differentiable functions f(x). where a is half the radius of convergence and c0,c1,c2,c3,c4. are coefficients.